Resonant Depolarization

Using a very strong kicker magnet the spin ensemble could be tilted into the horizontal plane during one pass through the kicker field, but without spin diffusion this does not lead to beam depolarization. However over many revolutions around the ring it is possible to tilt the mean spin vector bit by bit to the horizontal plane by using time-varying magnetic fields in resonance with the electron's spin revolutions. This procedure is depicted in figure 4.

Figure 4: For illustration: Resonant widening (half-integer resonance) of the precession cone by a time-varying magnetic kicker field. Because the electron is in this field only for a very short time (grey boxes) there are several resonant frequencies (depicted by the solid and dashed curves). ${\nu_0}^{-1}$ stands for the electron's revolution period around the ring, while ${\nu_{kick}}^{-1}$ is the period of the kicker magnet strength. [13]

Recalling equation 20 we can calculate the opening angle of the precession cone after 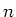$n$ revolutions:

$$\theta = n \cdot \overline{\Delta\theta}$$ (21)

and since we know that depolarization is reached by tilting the mean spin vector into the horizontal plane (over a time which is long enough to allow spin diffusion), we can define the depolarization time $\tau_{depol}$:

$$\theta = n \cdot \overline{\Delta\theta}$$

$$= n \cdot \left[\frac{2}{\pi}~\frac{ea}{m_ec}\cdot \left(B_{kick}\cdot l\right) \right]$$

$$= \tau_{depol} \cdot \nu_{0} \cdot \left[\frac{2}{\pi}~\frac{ea}{m_ec}\cdot \left(B_{kick}\cdot l\right) \right]$$

$$\stackrel{!}{=} \frac{\pi}{2}$$ (22)

and therefore, we derive:

$$\tau_{depol} = \frac{\pi}{2} \cdot \left[\frac{2}{\pi}~\frac... ...ft(B_{kick}\cdot l\right) \right]^{-1} \cdot \frac{1}{\nu_{0}}$$ (23)

In the case of the SLS storage ring this leads to:

$$\tau_{depol}\left[s\right] \approx 3.48 \cdot 10^{-6} \cdot \frac{1}{B_{kick} \cdot l\left[ Tm\right]}$$ (24)

If we require depolarization time not to be longer than a second it can be derived from equation 24:

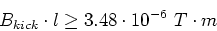$$B_{kick} \cdot l \ge 3.48 \cdot 10^{-6}~T \cdot m$$ (25)

Such values can be well achieved with the multi-bunch feedback kicker magnets installed at SLS ( $B \cdot l < 10^{-4}~T\cdot m$).